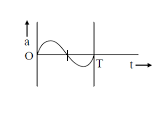
The oscillation of a body on a smooth horizontal surface is represented by the equation

⇦

⇨

 The oscillation of a body on a smooth horizontal surface is represented by the equation,
X=A cos(ωt)
where, X=displacement at time t
ω= frequency of oscillation
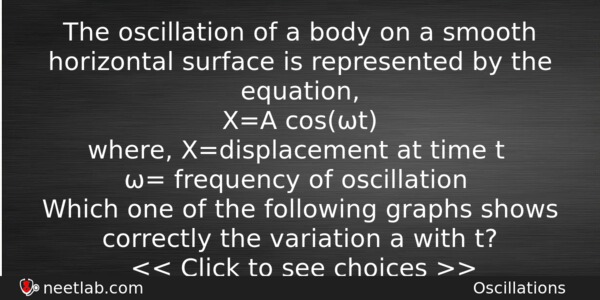
Which one of the following graphs shows correctly the variation a with t?

Options

(a)

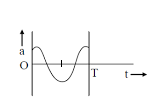
(b)

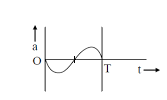
(c)

 

 

 

 

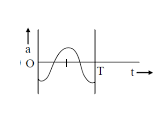
(d)

Correct Answer:

Explanation:

No explanation available. Be the first to write the explanation for this question by commenting below.

Related Questions:

1. If h is Planck’s constant, the momentum of a photon of wavelength 0.01 Å is
2. During an adiabatic process, the pressure of a gas is found to be proportional
3. Application of a forward bias to a P-N junction
4. A current of 2A flows through a 2Ω resistor when connected across a battery
5. An engine pumps water through a hose pipe. Water passes through the pipe

Topics: Oscillations (58)
Subject: Physics (2479)

---

Important MCQs Based on Medical Entrance Examinations To Improve Your NEET Score

18000+ students are using NEETLab to improve their score. What about you?

Solve Previous Year MCQs, Mock Tests, Topicwise Practice Tests, Identify Weak Topics, Formula Flash cards and much more is available in NEETLab Android App to improve your NEET score.

---

Share this page with your friends